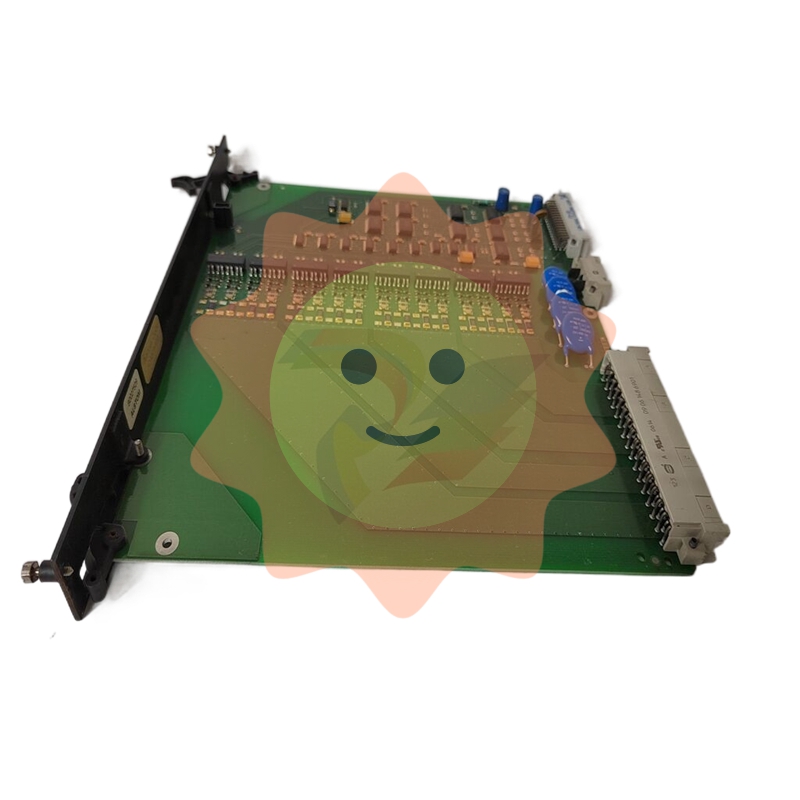
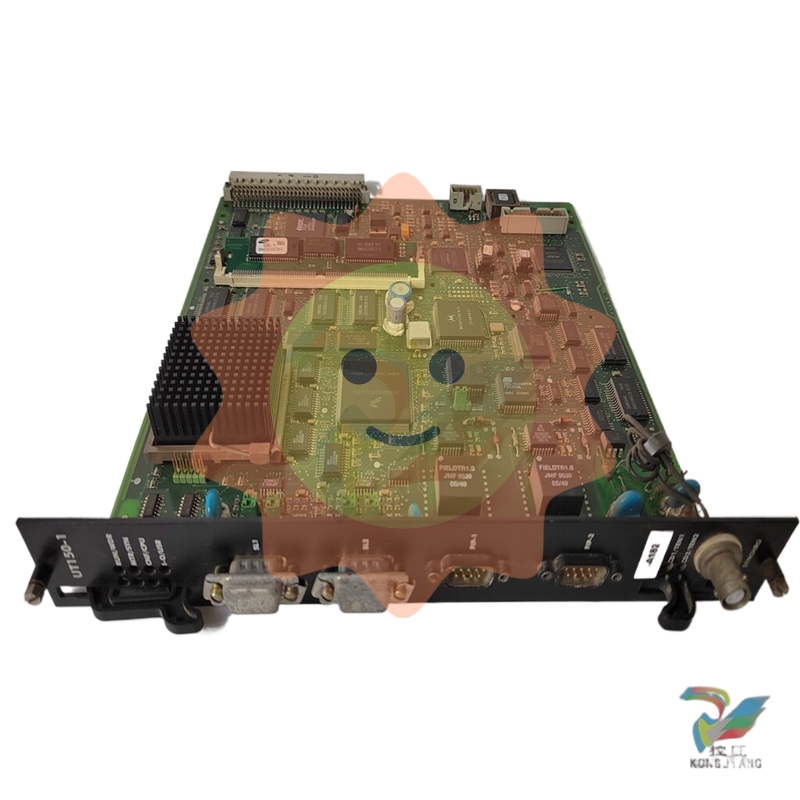
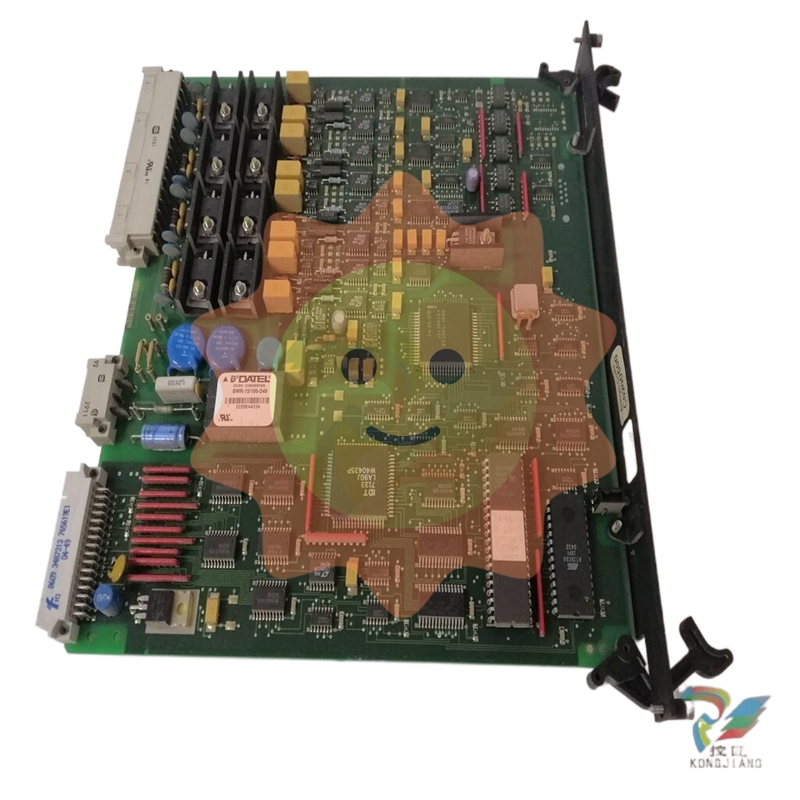
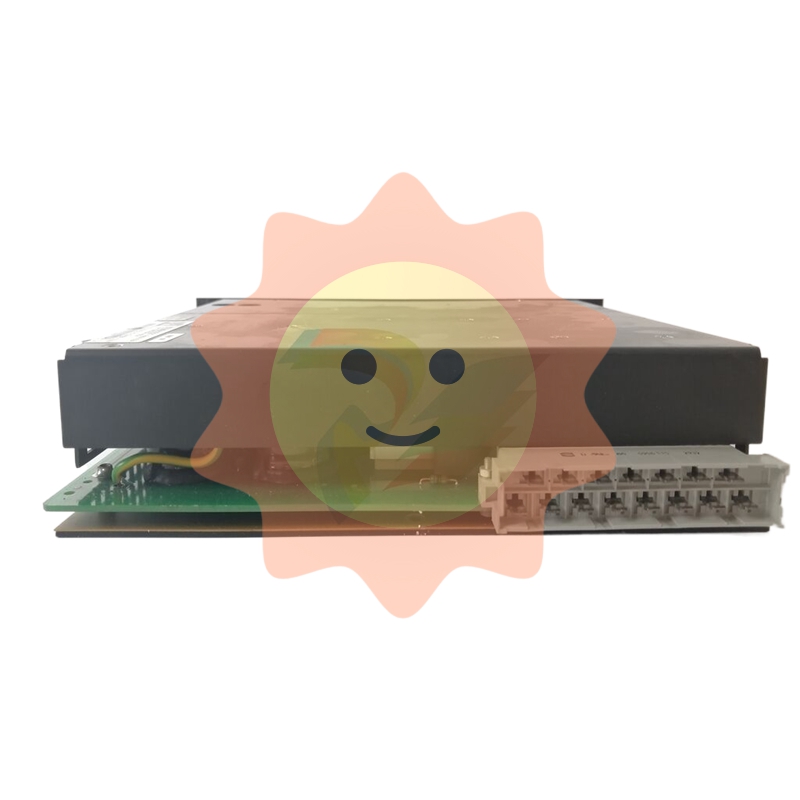
Electrons can "fission" and release photons to obtain recoil

Why electrons don't fall into the nucleus. So why don't electrons fall into the nucleus under electrostatic and magnetic forces? We know that nuclei and electrons at a certain distance will be close to each other along the helix under the action of electrostatic and magnetic forces, and the electrons will be deformed under the strong tearing action of the electrostatic attraction of the nucleus; When the distance between the electron and the nucleus is close enough, the electron will inevitably "fission" and release photons to obtain recoil and further increase the speed of the electron around the nucleus. At this time, due to the increase in the speed of the electron around the nucleus, the centrifugal trend will also increase, so the electron will move farther away from the nucleus, so the electron will not fall into the nucleus. When an electron in a stable orbit is disturbed by the external disturbance directed at the nucleus, for example, when we apply high pressure to a substance, it will inevitably force the electron to move close to the nucleus. At this time, the electron will be torn by more electrostatic force because it is closer to the nucleus. In order to "save the car", the electron will continue to fission and release photons to obtain recoil, thus increasing the speed of the electron moving around the nucleus. To continue to counter the electrostatic pull of the nucleus. In the macroscopic world, even if one charged particle is artificially spun around another charged particle at high speed to form an atomic-like system (although this is difficult to do), the atomic-like system formed by the macroscopic charged particle is very fragile, and the atomic-like system will fall apart whether the particle is subjected to the disturbance of the nucleus or the disturbance of the near nucleus. The main reason is that the mass of macroscopic charged particles does not change, and they do not change their mass to maintain the equilibrium of atom-like systems. Here we have to admire the wonder of the material world, a small atomic system in the microscopic world is much more advanced and stable than the atom-like system we force to form with macroscopic charged particles.

The movement of electrons in the nucleus. Let an electron with a mass of M rotate stably around the nucleus in an orbit with a distance of R from the nucleus (at this time, the electron must be in a "magic number" peak position with a large internal binding force), at this time, the "tearing action" of the nucleus's electrostatic gravity must be less than the internal binding force of the electron, and this balance will be maintained if it is not disturbed by the outside world. Under normal circumstances, electrons are always subject to external disturbances (such as collisions between atoms, collisions between photons and electrons, etc.). If an electron is impacted by a photon of mass m pointing to the nucleus at a certain moment, because the electron is always in a "hungry state" under the electrostatic gravity of the nucleus. Therefore, at the moment of the encounter between the photon and the electron, the electron will absorb the photon and increase its mass and move towards the nucleus. Assuming that the distance of the electron moving towards the nucleus is r, then the distance from the electron to the nucleus (the radius of the electron around the nucleus) is R-R, and the internal binding force of the electron mass is M+ m, due to the increase of the electron mass, will inevitably decrease rapidly. The reduction of the distance between the electron and the nucleus will inevitably lead to the rapid increase of the tearing effect of the electrostatic attraction of the nucleus on the electron. If the internal binding force of the electron is less than the tearing effect of the electrostatic attraction of the nucleus on the electron, the electron will quickly "fission" to release a mass m photon and get a recoil back to the original orbit farther from the nucleus. It has been suggested that an electron with a mass of M+ m does not give off photons of other masses after fission. This is because electrons in the mass interval of M+ m and M-m, there is only a maximum binding energy - the corresponding mass is M, in other words, only the internal binding force of the mass of m is large enough to resist the electrostatic gravitational tearing of the nucleus, and other masses of electrons are unstable.

If at some point the electron is disturbed by a photon of mass m moving away from the nucleus, since the electron is in a "hungry state", the electron will absorb the photon and increase its mass and move away from the nucleus at the moment the photon meets the electron. Assuming that the furthest distance of the electron from the nucleus is R+r and the mass of the electron is M+m, since the original electron is at the peak of the "magic number of mass" with the greatest internal binding force, the internal binding force will inevitably decrease rapidly after the absorption of the photon, and the increase of the distance of the electron from the nucleus will lead to the reduction of the electrostatic attraction of the nucleus on the electron. If an electron with a mass of M+m is located at another peak position where the internal binding force is larger, if the tearing effect of the nucleus's electrostatic attraction on the electron is less than the internal binding force, the electron will stabilize in a new orbit R+r away from the nucleus, and the electron will transition after being excited. If the mass of M+m electron is not located in the peak position of the internal binding force is large, then the internal binding force of the new mass of M+m electron will be much less than that of the original mass of M electron. If the tearing effect of the electrostatic attraction of the nucleus on the electron is greater than the internal binding force of the electron, the electron will also release the mass of m photon and return to the original orbit.